Kartesische Gleichung eines Flugzeugrechners

Point A , ,
Point B , ,
Point C , ,
   
Equation of the plane
(given three points)		 x+y+z+=0

Es gibt drei Punkte A (x 1 , y 1 , z 1 ), b (x 2 , y 2 , z 2 ) und c (x 3 , y 3 , z 3 ) Liegen in einer Ebene, dann kann die ebene Gleichung mit der folgenden Formel gefunden werden

.

.

x - x 1

y - y 1

z - z 1

= 0

x 2 - x 1

y 2 - y 1

z 2 - z 1

x 3 - x 1

y 3 - y 1

z 3 - z 1

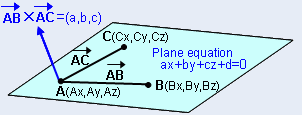
Oder a (Axt, ay, az), b (bx, von, bz), c (cx, cy, cz), die Gleichung der Ebene ist AX + durch + cz + d = 0

Wo,

A = (BY-AY) (CZ-AZ) - (CY-AY) (BZ-AZ)

B = (BZ-AZ) (CX-AX) - (CZ-AZ) (BX-AX)

C = (BX-AX) (CY-AY) - (CX-AX) (BY-AY)

d = - (AAX + BAY + CAZ).

Die kartesische Ebenengleichungsberechnung mit den drei Koordinaten wird hier erleichtert.

Kartesische Gleichung eines Flugzeugrechners
Empfehlung Taschenrechner Luftgeschwindigkeitslöser erfassen Gallonen pro Stunde Kraftstoffrechner Fließwandlerrechner Luftkanalflussrechner Wärmerückgewinnungsrechner Volumeneinheit-Konverter Drehmoment zum PS-Konverter Windleistungsrechner Hydraulikdurchmesser Solver. Rauschkriteriumrechner Geschwindigkeitsumrichter Luftkanal-Velocity-Rechner Luftstromkopfverlustrechner Kapazität des Erdgasrohrs Rechner Elektromotorenrechner